Poisson Distribution Calculator
Enter the average rate of success and the number of occurrences in the calculator below to find the probabilities of an event occurring using the Poisson distribution.
Probabilities:
| P(X = x): | |
|---|---|
| P(X < x): | |
| P(X ≤ x): | |
| P(X > x): | |
| P(X ≥ x): |
Steps to Solve
Probability Mass Function
f(x; λ) = P(X = x) = λx × e-λ / x!
Substitute Values in the Formula and Solve
The solution will be shown here after you enter values in the form above
On this page:
Joe is the creator of Inch Calculator and has over 20 years of experience in engineering and construction. He holds several degrees and certifications.
What is a Poisson Distribution?
In statistics, the Poisson distribution is a probability distribution that describes the probability of an event occurring a specified number of times in a particular time frame, assuming that the occurrence of one event gives you no information about whether the next event is going to come sooner or later. This is known as the Poisson probability.
Some situations where a Poisson distribution might be used might be modeling the number of calls to a doctor’s office in a particular time frame or the number of cars that travel on some segment of highway. Indeed, because of the situations where the Poisson distribution tends to be used, it is often said to model the number of “arrivals” in a particular time frame.
The Poisson distribution is a way of specifying the likelihood of a specific number of occurrences of an event happening within a period of time.
The Poisson distribution is a probability distribution, but unlike a normal distribution, it is a discrete function that can only take non-negative whole numbers. Fractions or decimal values are not supported.
Unlike a binomial distribution, which counts discrete occurrences among discrete trials (e.g. coin flips), a Poisson distribution counts discrete occurrences among a continuous domain (e.g. a period of time).
How to Calculate a Poisson Probability
You can find the probability of events occurring using the Poisson distribution using its probability mass function. In order to use the function described by the formula below, you need to know the average rate of success, or the average number of times the event occurs.
Poisson Distribution Formula
The probability of a specific number of occurrences of an event is described by the following formula:
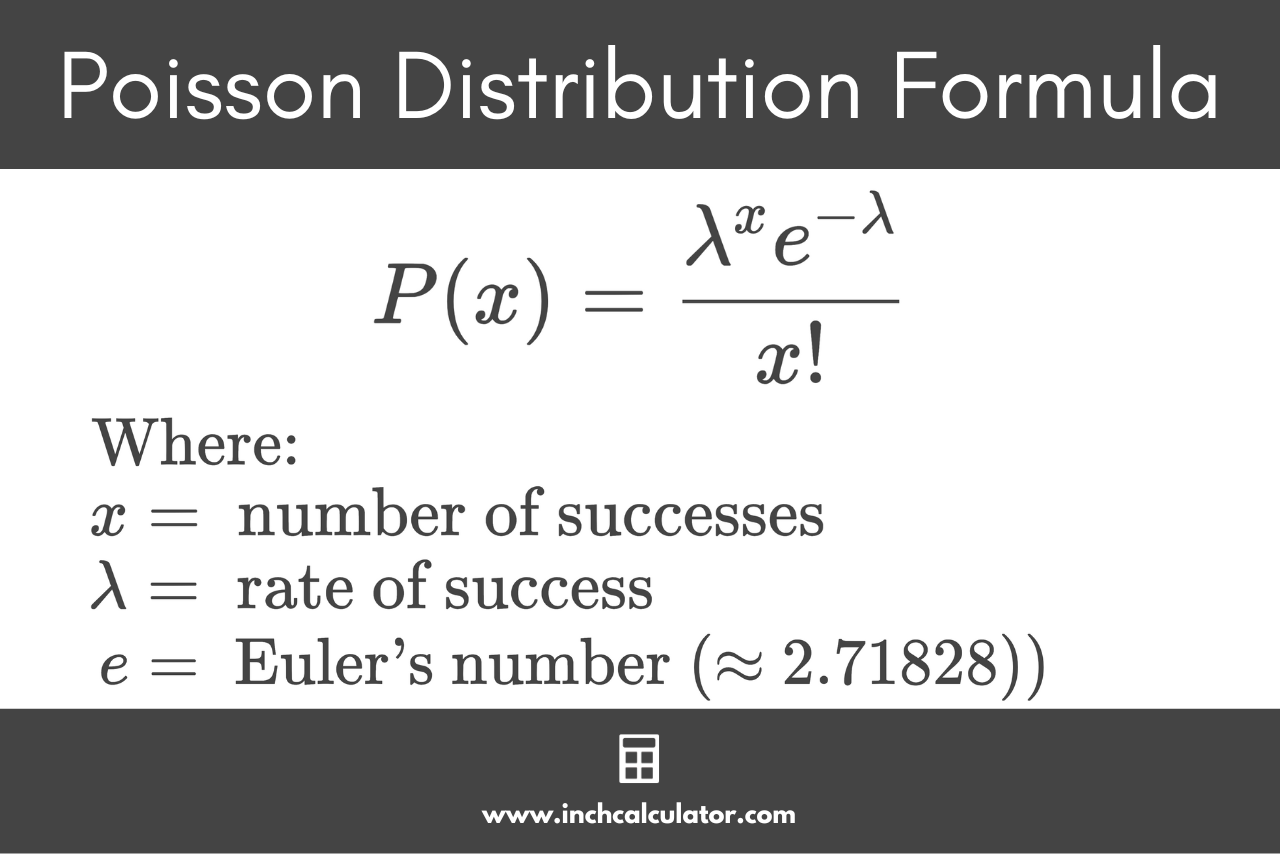
P(x) = λxe-λ / x!
Where:
- x is the number of occurrences (Poisson random variable)
- λ is the rate of success (Greek letter lambda)
- e ≈ 2.71828 (Euler’s number)
- both x and λ are non-negative integers
Thus, the probability of an event occurring x number of times is equal to the average rate of success λ to the power of x times the reciprocal of Euler’s number e to the power of λ, divided by x factorial.
You can use a factorial calculator or exponent calculator to help solve those parts of the formula.
For example, suppose that, on average, four people call a doctor’s office to make an appointment on a weekday morning. Let’s find the probability that 7 will call on some day.
Let’s start by substituting the average rate (λ) of 4 and the number of occurrences (x) of 7 in the formula above, then solve.
P(X = 7) = 47 × e-4 / 7!
P(X = 7) = 47 × 2.71828-4 / 7 × 6 × 5 × 4 × 3 × 2 × 1
P(X = 7) = 16,384 × 0.01831564 / 5,040
P(X = 7) = 300.08342755 / 5,040
P(X = 7) = 0.0595
Thus, the probability of exactly 7 people calling this doctor’s office on some weekday morning is 0.0595, or a 5.95% chance.