Amps to Watts Conversion Calculator
Enter the current and voltage to convert amps to watts for DC and AC single and three-phase circuits.
Power:
On this page:
- Amps to Watts Calculator
- How to Convert Amps to Watts
- Amps to Watts Formula
- How Many Watts Are in an Amp?
- Conversion for Single-Phase AC Circuits
- Conversion for Three-Phase AC Circuits
- How to Convert Amps and Ohms to Watts
- Table: Amps to Watts at 120V & 240V AC
- Table: Amps to Watts at 12V DC
- References
Joe is the creator of Inch Calculator and has over 20 years of experience in engineering and construction. He holds several degrees and certifications.
Aditya holds a PhD in electrical engineering from Stanford University and specializes in signal processing, algorithms, and machine learning.
Kevin Weekly holds a PhD in Electrical Engineering and Computer Science (EECS) from the University of California Berkeley and Bachelor's degrees in electrical engineering and computer science from the University of Texas.
How to Convert Amps to Watts
Converting amps to watts can be done using the Watt’s Law power formula, which states that P = I × V, where P is the power measured in watts, I is the current measured in amps, and V is the voltage measured in volts.
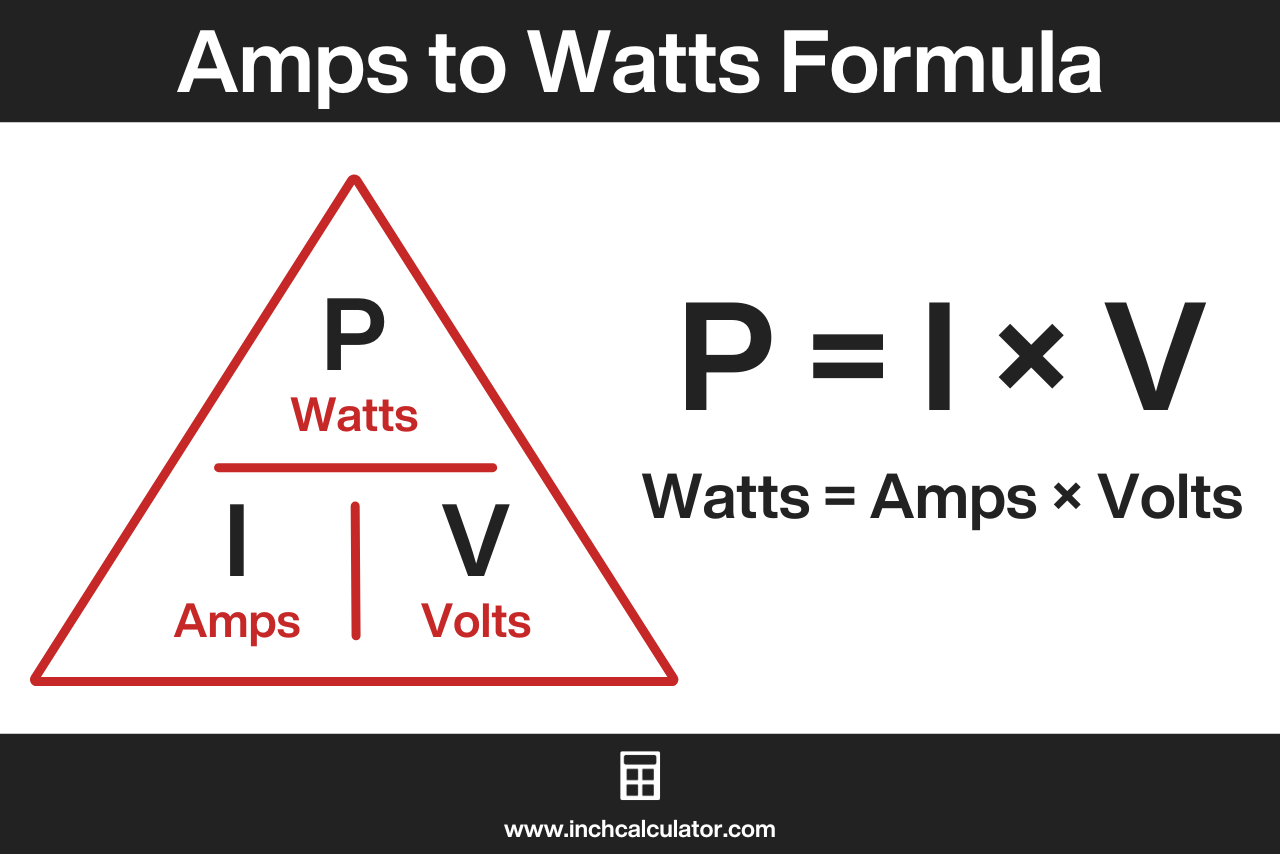
Amps to Watts Formula
Given the current and voltage, it is possible to compute the power in watts using the following formula:[1]
P(W) = I(A) × V(V)
Thus, the power P in watts is equal to the product of the current I in amps and the voltage V in volts.
How Many Watts Are in an Amp?
Using the formula above, you can also figure out how many watts of power there are in one amp, depending on the circuit voltage. For example, at 120 volts, 120 watts of power are consumed at 1 amp, and 240 watts are consumed at 2 amps.
Likewise, at 240 volts, 240 watts of power are consumed at 1 amp, and 480 watts are consumed at 2 amps.
Example: 15 Amps to Watts
For example, let’s calculate the wattage capacity of a 15-amp, 120-volt electrical circuit.
P(W) = 15 A × 120 V
P(W) = 1,800 W
So, 15 amps of current at 120 volts will generate 1,800 watts of power.
Conversion for Single-Phase AC Circuits
Converting amps to watts for a single-phase AC circuit with a power factor requires a slight variation of the formula.
P(W) = I(A) × V(V) × PF
The power P in watts is equal to the product of the current I in amps, the voltage V in volts, and the power factor PF.
AC electrical power is composed of a real part expressed in watts and a reactive part expressed in volt-amps. The magnitude of both real and reactive together is called the apparent power, and the PF gives the ratio of real power to apparent power.[2]
The power factor is determined by the alternating current frequency and the inductive or capacitive elements in the circuit.
Try using our power factor calculator to find the PF value from power, current, and voltage.
Conversion for Three-Phase AC Circuits
The formulas to convert amps to watts for three-phase AC circuits are a bit different from the formulas above.
Using Line-to-Line Voltage
For three-phase AC circuits where the current, line-to-line RMS voltage, and power factor are known, the formula to convert amps to watts is:
P(W) = I(A) × VL-L(V) × PF × √3
The power P in watts is equal to the product of the current I in amps, the line-to-line voltage V in volts, the power factor PF, and the square root of 3.
This equation calculates the power for one pair of wires in a three-phase system, but you will need to multiply it by three when considering all three pairs of wires in a three-phase system.
Using Line-to-Neutral Voltage
For three-phase AC circuits where the current, line-to-neutral RMS voltage, and power factor are known, the formula to convert amps to watts is:
P(W) = I(A) × VL-N(V) × PF × 3
The power P in watts is equal to the product of the current I in amps, the line-to-neutral voltage V in volts, and the power factor PF, multiplied by 3.
This formula calculates the power delivered by all three wires in a three-phase system, but you will need to divide by three if you are considering a single wire in the three-phase system.
How to Convert Amps and Ohms to Watts
You can also convert amps to watts using circuit resistance with this formula:[1]
P(W) = I(A)2 × R(Ω)
The power P in watts is equal to the product of the square of the current I in amps and the resistance R in ohms.
Since 1 kilowatt is equal to 1,000 watts, it is possible to use the formulas above to also convert amps to kW, but the result will need to be divided by 1,000. You can also use our amps to kW calculator to solve for kilowatts.
Table: Amps to Watts at 120V & 240V AC
| Current (Amps) | Power (Watts) | Voltage (Volts) |
|---|---|---|
| 1 amp | 120 watts | 120 volts |
| 2 amps | 240 watts | 120 volts |
| 3 amps | 360 watts | 120 volts |
| 4 amps | 480 watts | 120 volts |
| 5 amps | 600 watts | 120 volts |
| 6 amps | 720 watts | 120 volts |
| 7 amps | 840 watts | 120 volts |
| 8 amps | 960 watts | 120 volts |
| 9 amps | 1,080 watts | 120 volts |
| 10 amps | 1,200 watts | 120 volts |
| 11 amps | 1,320 watts | 120 volts |
| 12 amps | 1,440 watts | 120 volts |
| 13 amps | 1,560 watts | 120 volts |
| 14 amps | 1,680 watts | 120 volts |
| 15 amps | 1,800 watts | 120 volts |
| 20 amps | 2,400 watts | 120 volts |
| 25 amps | 3,000 watts | 120 volts |
| 30 amps | 3,600 watts | 120 volts |
| 15 amps | 3,600 watts | 240 volts |
| 20 amps | 4,800 watts | 240 volts |
| 30 amps | 7,200 watts | 240 volts |
| 40 amps | 9,600 watts | 240 volts |
| 50 amps | 12,000 watts | 240 volts |
| 60 amps | 14,400 watts | 240 volts |
| 70 amps | 16,800 watts | 240 volts |
| 80 amps | 19,200 watts | 240 volts |
| 90 amps | 21,600 watts | 240 volts |
| 100 amps | 24,000 watts | 240 volts |
| 125 amps | 30,000 watts | 240 volts |
| 150 amps | 36,000 watts | 240 volts |
| 200 amps | 48,000 watts | 240 volts |
Table: Amps to Watts at 12V DC
| Current (Amps) | Power (Watts) | Voltage (Volts) |
|---|---|---|
| 1 amp | 12 watts | 12 volts |
| 2 amps | 24 watts | 12 volts |
| 3 amps | 36 watts | 12 volts |
| 4 amps | 48 watts | 12 volts |
| 5 amps | 60 watts | 12 volts |
| 6 amps | 72 watts | 12 volts |
| 7 amps | 84 watts | 12 volts |
| 8 amps | 96 watts | 12 volts |
| 9 amps | 108 watts | 12 volts |
| 10 amps | 120 watts | 12 volts |
| 11 amps | 132 watts | 12 volts |
| 12 amps | 144 watts | 12 volts |
| 13 amps | 156 watts | 12 volts |
| 14 amps | 168 watts | 12 volts |
| 15 amps | 180 watts | 12 volts |
| 20 amps | 240 watts | 12 volts |
| 30 amps | 360 watts | 12 volts |
| 40 amps | 480 watts | 12 volts |
| 50 amps | 600 watts | 12 volts |
| 60 amps | 720 watts | 12 volts |
| 70 amps | 840 watts | 12 volts |
| 80 amps | 960 watts | 12 volts |
| 90 amps | 1,080 watts | 12 volts |
| 100 amps | 1,200 watts | 12 volts |
References
- Miller, C., NFPA's Electrical References, National Fire Protection Association, 2004, Jones & Bartlett Learning, 67-75. https://www.google.com/books/edition/NFPA_s_Electrical_References/raUyIi7i-asC
- Fiore, J., AC Electrical Circuit Analysis - A Practical Approach, 2022, 274. http://www.dissidents.com/resources/ACElectricalCircuitAnalysis.pdf