Subtracting Fractions Calculator
Subtract one fraction from another by entering them below. You can optionally add a whole number to subtract a mixed fraction as well.
Result as a Fraction:
Result as a Decimal:
Steps to Solve Expression with Fractions
On this page:
Joe is the creator of Inch Calculator and has over 20 years of experience in engineering and construction. He holds several degrees and certifications.
Bailey is an experienced educator and specializes in K-12 math and science. She holds a Master's degree in professional education and holds several teaching certifications.
How to Subtract Fractions
A fraction is a numerical value that is not a whole number, and it generally represents a portion of a whole (e.g. one slice of a pie).
Subtracting fractions is a bit different from subtracting regular whole numbers, but you can use a few methods to do it.
Fraction Subtraction Formula
You can use the following formula to subtract fractions.
a / b – c / d = ad – bc / bd
To subtract two fractions, you can multiply the numerator of the first fraction with the denominator of the second fraction. Then, subtract it from the product of the numerator of the second fraction with the denominator of the first fraction, and write the result over the bottom of both fractions.
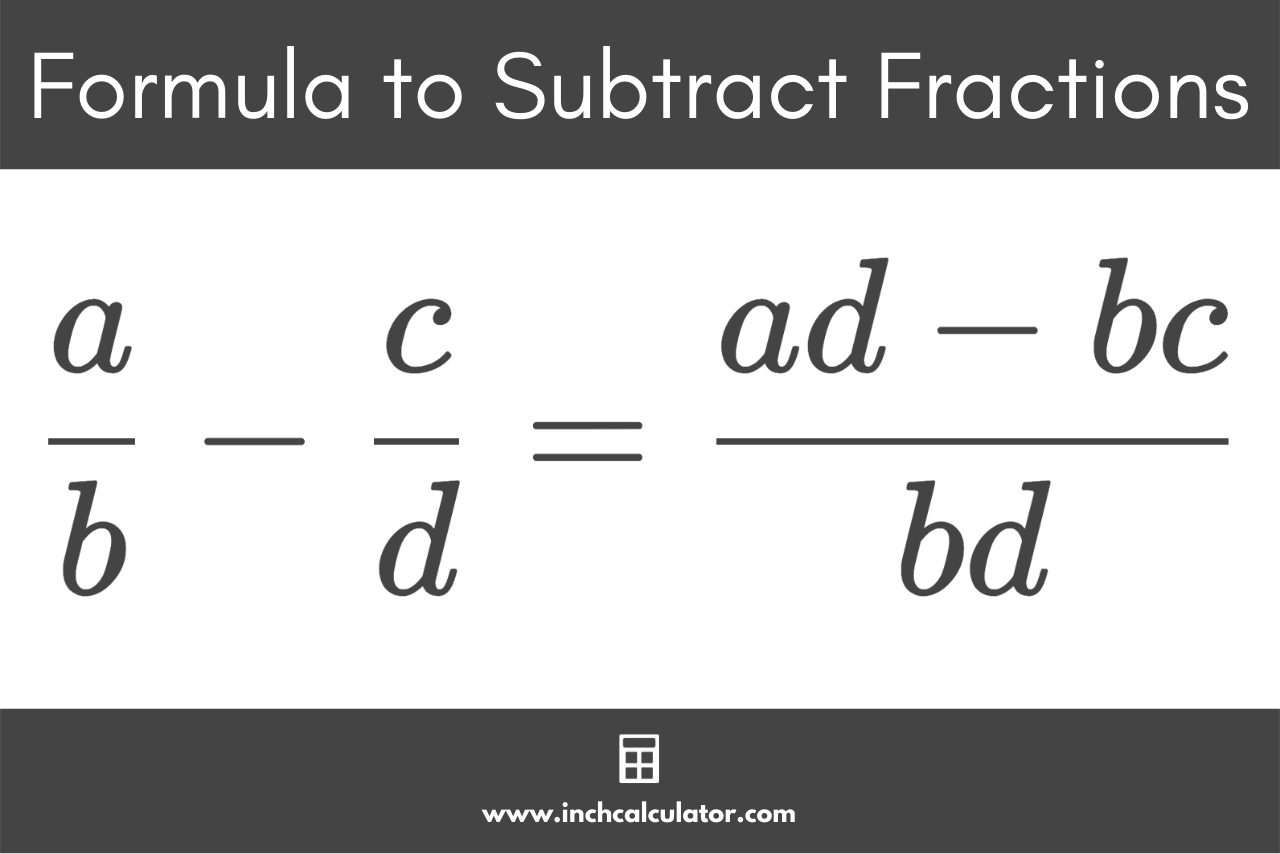
By multiplying the denominators together, The formula ensures that both denominators match and are both raised to a common denominator by multiplying them together.
Since the numerators are also multiplied using the same multiple as the denominator, the result is an equivalent fraction.
Then, the resulting numerators can be subtracted using normal means.
You can put this formula to practice and subtract fractions in three easy steps.
Step One: Convert to Fractions with a Common Denominator
The first step to subtracting fractions is to make the denominators of each fraction match. Convert each one to an equivalent fraction with a matching denominator to the other.
Find the Lowest Common Denominator
You’ll need to find the lowest common denominator for each of the fraction’s denominators. The lowest common denominator is the smallest number that each denominator can evenly divide into.
Find the Multiplier
Next, find the multiple for each denominator that can be multiplied to equal the lowest common denominator. To find the multiple for each fraction, divide the least common denominator by the denominator.
Multiply by the Multiplier
Then, multiply both the top and bottom number of the fraction by the multiple to find the equivalent fractions with matching denominators. You can also use an equivalent fractions calculator to find one with a matching denominator.
For example, let’s convert the fractions 1 / 2 and 1 / 3 to fractions with the same denominator.
The least common denominator of 2 and 3 is 6.
Find the multiple for 1 / 2
multiple = lcd ÷ denominator
multiple = 6 ÷ 2 = 3
Find the equivalent fraction of 1 / 2 using the multiple 3
1 / 2 = 1 × 3 / 2 × 3
1 / 2 = 3 / 6
Find the multiple for 1 / 4
multiple = lcd ÷ denominator
multiple = 6 ÷ 3 = 2
Find the equivalent fraction of 1 / 3using the multiple 2
1 / 3 = 1 × 2 / 3 × 2
1 / 3 = 2 / 6
Thus, the equivalent fractions of 1 / 2 and 1 / 3 are 3 / 6 and 2 / 6
Step Two: Subtract the Numerators
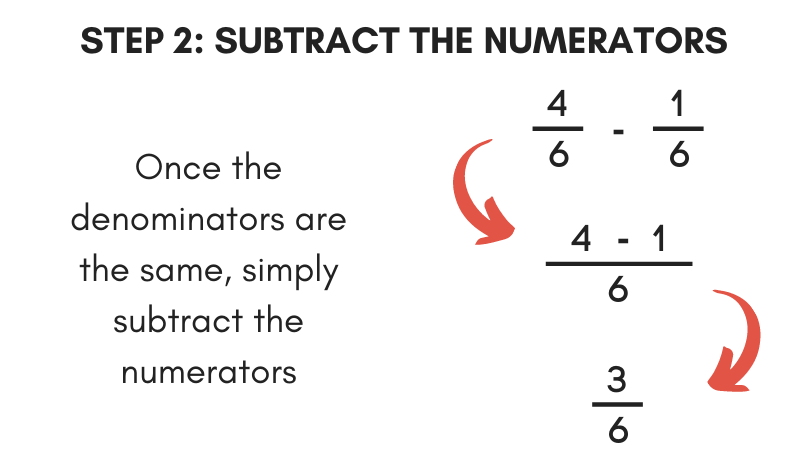
Once the bottom numbers of each fraction match, subtract the top numbers from each together to find the result.
Simply subtract the second numerator from the first, then put the result over the common denominator.
For example, let’s continue the previous example and subtract 2 / 6 from 3 / 6.
3 / 6 – 2 / 6 = 3-2 / 6
3 / 6 – 2 / 6 = 1 / 6
Step Three: Simplify the Fraction
The final step to subtracting fractions is to reduce the result to its simplest form. Start by finding the greatest common factor of the top and bottom parts of the result.
Then, divide both the top and bottom numbers by the greatest common factor to reduce it. An even easier way to reduce is to use our fraction simplifier.
You can also subtract fractions using our fraction calculator.
Frequently Asked Questions
Can you subtract fractions and decimals?
Subtraction of fractions and decimals is possible, but the numbers must first be converted to a common format. This can be done by converting fractions to decimals or vice versa, or by converting them to a common denominator or decimal place value before subtracting them.
What are the three rules to subtract fractions?
To subtract fractions, you need to make the denominators match by finding the least common multiple (LCM), subtract the numerators, and simplify the result if possible by finding the greatest common factor (GCF) and divide both numerator and denominator by it. Always check if the result is already in its lowest terms before simplifying.
Why can't you subtract the denominators of fractions?
You can’t subtract the denominators of fractions due to the fact that denominators represent the total number of equal parts that make up a whole, and are not always the same size between two fractions.