Terminal Velocity Calculator

Use our terminal velocity calculator to find the terminal velocity of a falling object.

Terminal Velocity:

Terminal Velocity Formula

v_{t}=\sqrt{\frac{2mg}{\rho AC_{d}}}

Learn how we calculated this below

How to Calculate Terminal Velocity

Terminal velocity is the highest velocity attainable by an object as it falls through a fluid (air, water, etc.). It occurs when the downward force of gravity (F_g) equals the upward force of drag (F_d).

This results in a net force of zero, causing the object to cease accelerating and maintain a constant velocity.

Several factors contribute to an object’s terminal velocity, including its mass, its cross-sectional area, its drag coefficient, and the density of the fluid through which it is falling.

Terminal Velocity Equation

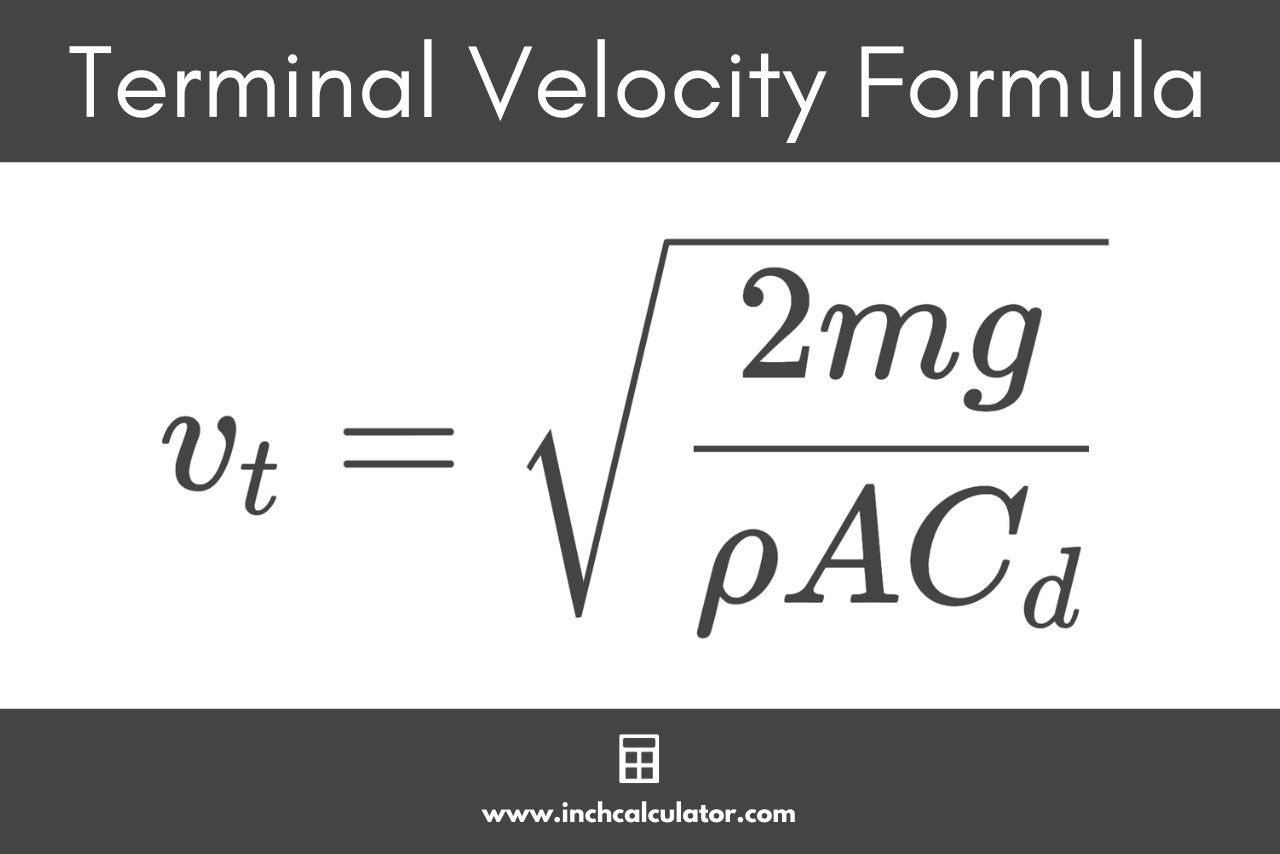
The formula for terminal velocity v_t can be expressed as:

v_{t}=\sqrt{\frac{2mg}{\rho AC_{d}}}

Where:
m = mass of the falling object
g = acceleration due to gravity
ρ = fluid density
C_d = drag coefficient
A = cross-sectional area of the object

The terminal velocity formula states that the terminal velocity of a falling object v_t is equal to the square root of 2 times the object’s mass m times the gravitational acceleration g divided by the fluid density ρ times the object’s drag coefficient C_d times the object’s cross-sectional area A.

Graphic showing the terminal velocity formula.

Derivation of the Terminal Velocity Formula

The derivation of the terminal velocity formula begins with Newton’s second law of motion, which states that the net force acting on an object is equal to the mass of the object multiplied by its acceleration (F = ma).

For an object falling through a fluid, two primary forces act: gravitational force (F_g) and drag force (F_d). The gravitational force is given by:

F_{g}=mg

The drag force can be described by the formula:

F_{d}=\frac{1}{2}\rho C_{d}Av^{2}

At terminal velocity, the acceleration of the object is zero because the net force is zero. Thus, setting F_g = F_d:

mg=\frac{1}{2}\rho C_{d}Av_{t}^{2}

Solving for v_t gives us:

v_{t}=\sqrt{\frac{2mg}{\rho AC_{d}}}

For example, let’s consider a scenario where we need to calculate the terminal velocity of a skydiver.

Let’s assume the skydiver’s mass is 80 kg, the drag coefficient is 1.0 (body in freefall position), the cross-sectional area is 0.7 m², and the air density at the altitude of the jump is approximately 1.2 kg/m³. The acceleration due to gravity is about 9.81 m/s².

v_{t}=\sqrt{\frac{2 \cdot 80 kg \cdot 9.81 m/s²}{1.2 kg/m³ \cdot 0.7 m² \cdot 1.0}}

v_{t}=\sqrt{\frac{1569.6}{0.84}}

v_{t}=\sqrt{1868.57}

v_{t}=43.2\ m/s

So, the terminal velocity of the skydiver in this example is about 43.2 meters per second.

Terminal Velocity Calculator

Have a Question or Feedback?