Exponential Growth Calculator
Use our exponential growth calculator to find the final value, initial value, growth rate, or time.
Final Value:
Joe is the creator of Inch Calculator and has over 20 years of experience in engineering and construction. He holds several degrees and certifications.
Aditya holds a PhD in electrical engineering from Stanford University and specializes in signal processing, algorithms, and machine learning.
How to Calculate Exponential Growth
Exponential growth is the growth of a value by the same percentage over a number of time periods, where the percentage of growth during each period includes the effect of growth accumulated over previous periods.
Exponential growth is the mechanism behind things such as compound interest (positive exponential growth) and half-life decay (negative exponential growth, or decay).
You can calculate the amount of growth in a value over time using the exponential growth formula.
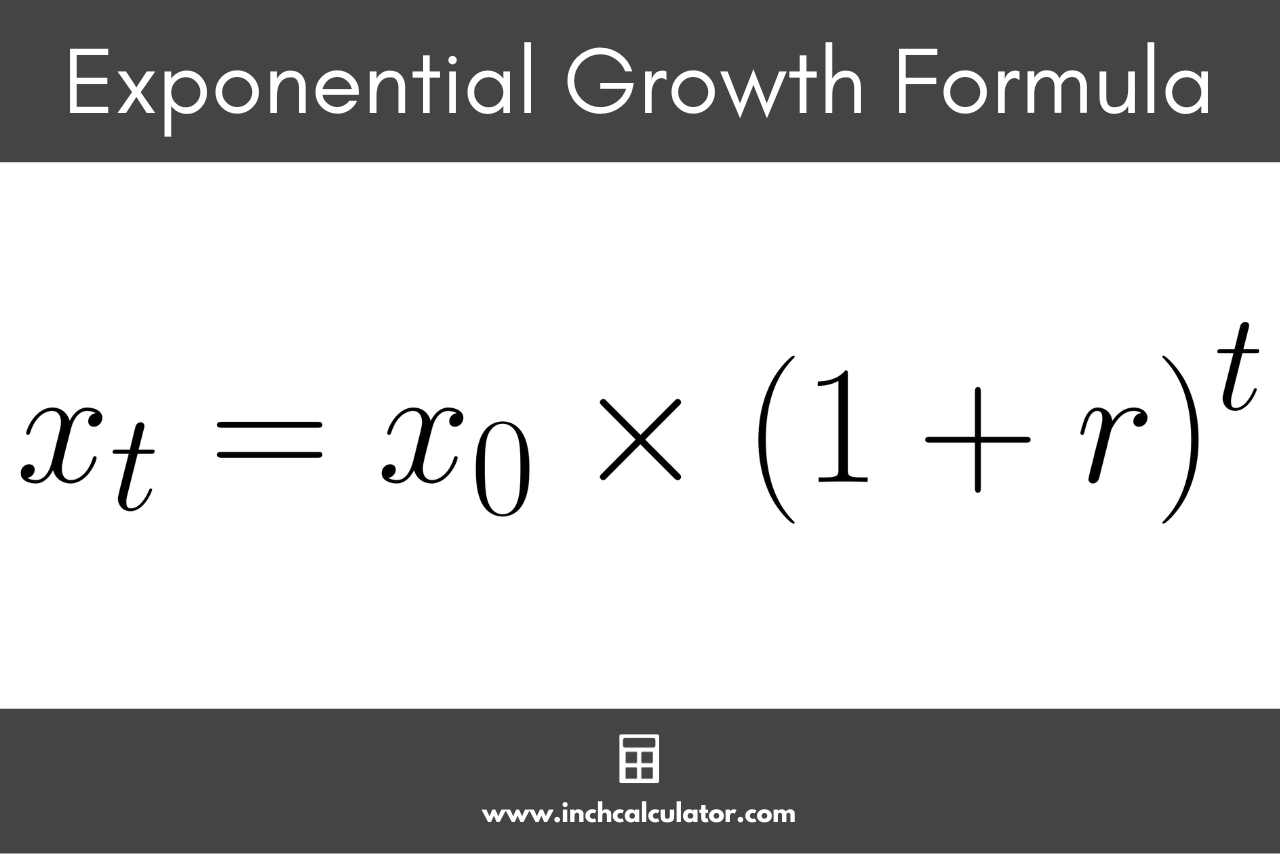
Exponential Growth Formula
The formula to find a value using the exponential growth formula is:
The final value xt is equal to the initial value x0 times 1 plus the growth rate r raised to the power of the number of time periods t.
By using a negative growth rate, you can also calculate the amount of exponential decay in a value.
The initial value grows over time if growth rate r > 0 and decreases (decays) over time if r < 0.
For example, let’s calculate the growth in a savings account containing $100 that grows at a rate of 7% over 20 years.
So, a $100 savings account that grows exponentially at a rate of 7% per year over 20 years will have a final value of $386.97.