Binary to Decimal Converter

Enter a binary number below to convert it to a decimal.

Decimal Number:

Steps to Convert to Decimal

Learn how we calculated this below

How to Convert Binary to Decimal

The binary number system is a base 2 number system since it only uses the digits 0 and 1, unlike the decimal number system, which is a base 10 number system since it uses ten digits, 0 to 9.

Often you will need to convert a binary number to its decimal value since the decimal system is used most often. Binary numbers are often used in computing applications.

To convert a binary number to a decimal, you can use the positional notation method. To use this method, multiply each digit in the binary number from the rightmost number to the left by 2 to the power of n, where n is the distance from the right.

So, reading the binary number from right to left, the furthest digit to the right is equal to the digit times 2⁰. The digit that is one position from the right is equal to the digit times 2¹.

Binary to Decimal Formula

Thus, the binary to decimal formula is:

decimal number₁₀ = (d₀ × 2⁰) + (d₁ × 2¹) + … + (d_{n – 1} × 2^{n – 1})

In this formula, d₀ is the binary digit furthest to the right, d₁ is the digit one position from the right, and d_{n – 1} is the digit furthest to the left.

graphic showing how to convert a binary number to decimal

You can also use a tool like our binary calculator to convert to decimal or hex.

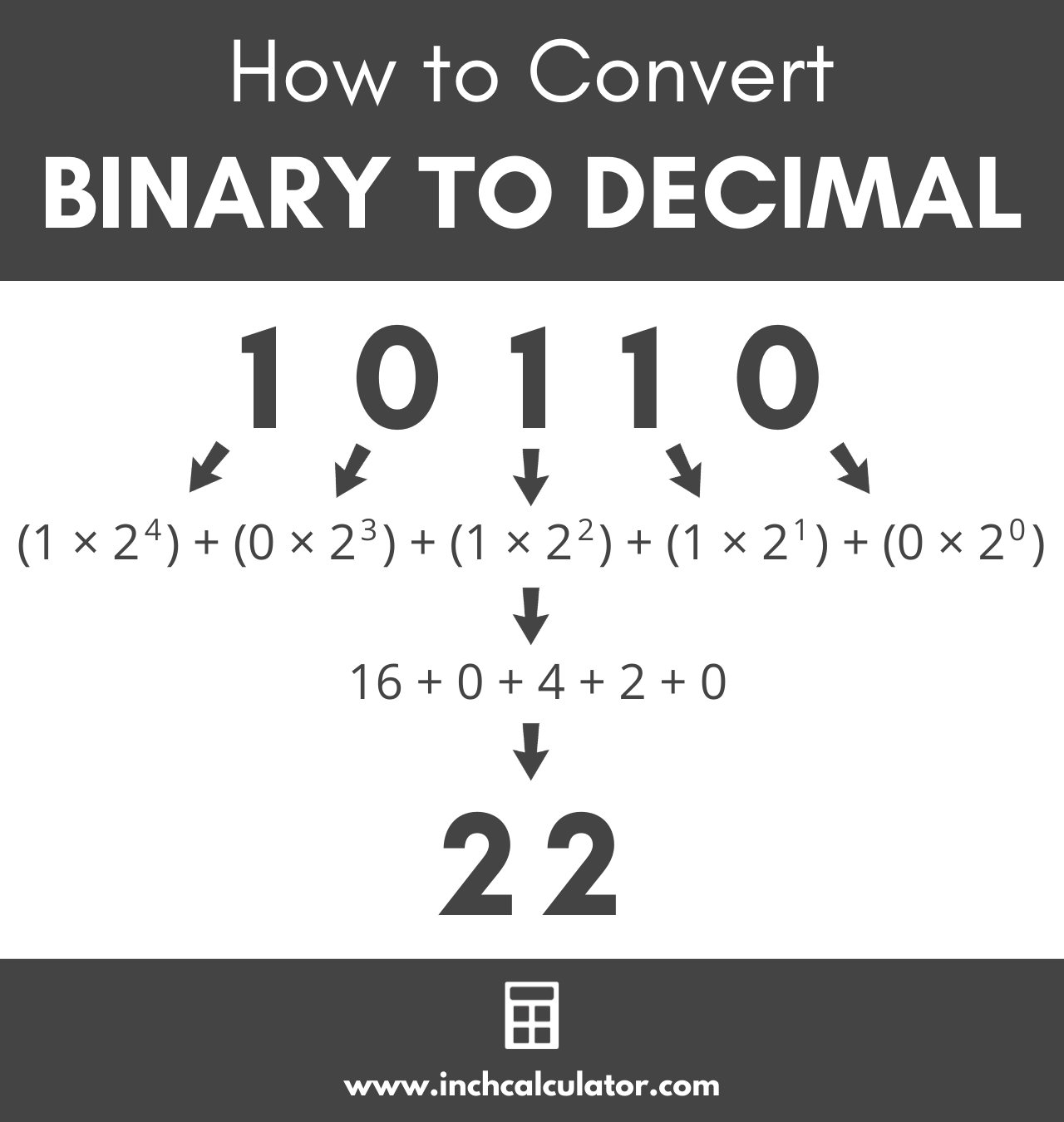
For Example, let’s convert the binary number 10110 to decimal.

decimal number₁₀ = (1 × 2⁴) + (0 × 2³) + (1 × 2²) + (1 × 2¹) + (0 × 2⁰)
decimal number₁₀ = 22

Binary to Decimal Conversion Video Tutorial

How to Convert Fractional Binary Numbers to Decimal

For real numbers that contain fractional values, you can use the positional notation system to convert as well. Start by using the method above to convert the whole portion of the number from binary to decimal.

Then, for the remaining fractional value, multiply each number to the right of the decimal point by 2 to the power of -1 times the distance from the decimal point plus 1. That sounds complicated, but it’s easier than it sounds; let’s demonstrate.

decimal number₁₀ = (d_-1 × 2^-1) + (d_-2 × 2^-2) + … + (d_-n × 2^-n)

For Example, let’s convert the binary number 0.101 to decimal.

decimal number₁₀ = (1 × 2^-1) + (0 × 2^-2) + (1 × 2^-3)
decimal number₁₀ = (1 ÷ 2¹) + (0 ÷ 2²) + (1 ÷ 2³)
decimal number₁₀ = 0.5 + 0 + 0.125
decimal number₁₀ = 0.625

The calculator above can convert binary numbers to decimals, including fractional numbers like this one, along with negative numbers.

Binary to Decimal Conversion Table

The table below shows binary numbers and the equivalent decimal number values.

Binary numbers converted to the equivalent decimal values
Binary Number	Decimal Number
0	0
1	1
10	2
11	3
100	4
101	5
110	6
111	7
1000	8
1001	9
1010	10
1011	11
1100	12
1101	13
1110	14
1111	15
10000	16
10001	17
10010	18
10011	19
10100	20
10101	21
10110	22
10111	23
11000	24
11001	25
11010	26
11011	27
11100	28
11101	29
11110	30
11111	31
100000	32
1000000	64
10000000	128
100000000	256
1000000000	512
10000000000	1024
100000000000	2048

Frequently Asked Questions

Why does binary go from right to left?

Binary goes from right to left because the lowest weighted are on the right and the highest weighted are on the left.

When would you convert from binary to decimal?

You would convert from binary to decimals when you need the information to be more readable by humans or when the value needs to be used in non-computing applications.

Why can't computers use decimal numbers?

Computers don’t use decimal numbers because they are built upon transistors that support two states, on and off. If computers used decimal numbers, they would need to support ten states.

In addition, many mathematical operations, such as multiplication and division, are simpler to perform in binary, so using the decimal system would require them to work much harder to process information.