Net Present Value Calculator
Calculate the net present value of an investment using our NPV calculator below.
Results:
| Net Present Value (NPV): | $ |
| Net Cash Flow (NCF): | $ |
| Return on Investment (ROI): | %
|
| Internal Rate of Return (IRR): | %
|
On this page:
Joseph Rich holds a Master's degree in finance and a Bachelor's degree in economics. He specializes in economics and investing analysis.
Monica Greer holds a PhD in economics, a Master's in economics, and a Bachelor's in finance. She is currently a senior quantitative analyst and has published two books on cost modeling.
Laura started her career in Finance a decade ago and provides strategic financial management consulting. She has an MBA in Finance and a Bachelor's in Economics.
How to Calculate Net Present Value
Net present value (NPV) is a calculation used in corporate finance and capital budgeting to determine whether a company should invest in a particular project. It is the future cash flows of an investment discounted to the present value of it today.
Anytime the NPV of a project is greater than $0, the company should invest in it. Anytime a project’s NPV is below $0, the company should avoid investing in it.
Net Present Value Formula
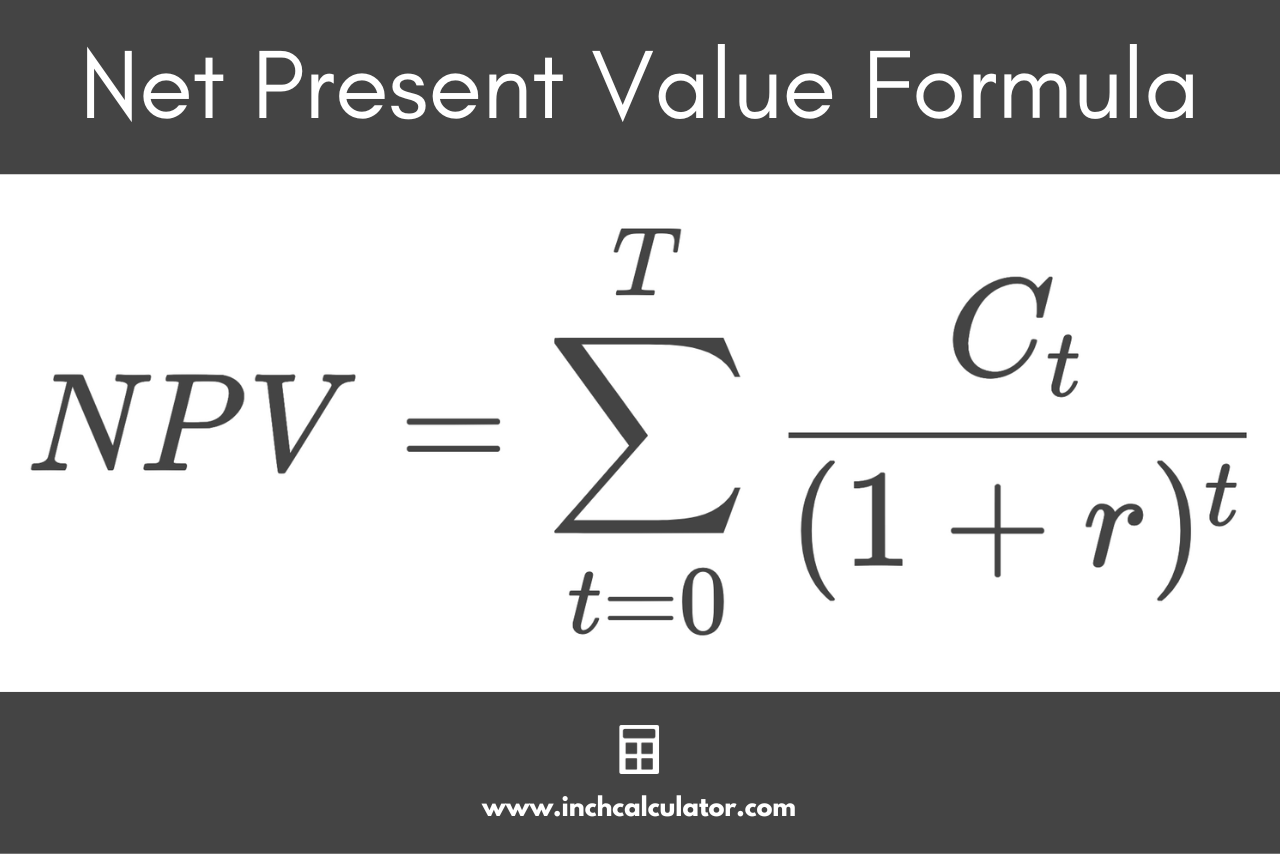
The NPV formula is shown below. To use this formula, the company must be able to estimate the cash flows the project will generate each year as well as the number of years. The discount rate is the rate that has been used for projects with similar risks.
where:
NPV = net present value
Ct = cash flow for a given period
t = time period
r = discount rate
Thus, the net present value is equal to the sum of the cash flows Ct for each period divided by the discount rate r plus 1, to the power of the time period t.
Since this formula uses the sum function, it is easiest to do with a calculator, like the one that is provided above, especially if there are many periods. But we can still show how it can be manually calculated using the table below.
For example, let’s say a company is deciding whether or not it should invest in a project that requires an initial $5,000 cash outflow followed by cash inflows of $1,300 each year for the next 5 years. Given a discount rate of 10%, let’s calculate what the NPV would be
By plugging these amounts into the calculator above, we get an NPV of -$71.98. Since this is a negative number, the company would not want to move forward with the project. The table below shows how you can manually calculate this amount.
| Time | Cash Flow | Discounted Cash Flow |
|---|---|---|
| 0 | $(5,000.00) | $(5,000.00) |
| 1 | $1,300.00 | $1,181.82 |
| 2 | $1,300.00 | $1,074.38 |
| 3 | $1,300.00 | $976.71 |
| 4 | $1,300.00 | $887.92 |
| 5 | $1,300.00 | $807.20 |
| Sum | -$71.98 |
The column on the right is calculated by taking the cash flow (middle column) for each time period and dividing by (1+10%)t. The right column is then summed up to arrive at -$71.98. This is exactly what the NPV formula above states.
Using an NPV or IRR calculator, you can see that the IRR (internal rate of return) is 9.44%. This means that any discount rate greater than 9.44% will lead to a negative NPV. Any discount rate below 9.44% will lead to a positive NPV.
If you enter 9%, as opposed to 10%, for the discount rate, we now get a positive net present value of $56.55.
Also, if you enter 9.44% for the discount rate, the NPV is $0.
The calculator above shows two other amounts as well: the net cash flow of $1,500 and the return on investment (ROI) of 30%.
The net cash flow is found by summing all the cash flows and subtracting the initial investment. In our example, we had $6,500 in cash flows ($1,300 x 5) and $5,000 as the initial investment. By subtracting $5,000 from $6,500, we get net cash flows of $1,500.
The ROI is calculated by taking the net cash flows and dividing by the initial investment. Dividing $1,500 by $5,000 yields an ROI of 30%.
What is the Difference Between Present Value (PV) and NPV?
Present value and net present value are similar. Present value is the amount needed to be invested today in order to earn a certain amount in the future. Net present value is the difference between an initial investment and cash inflows over a period of time.
Let’s go through an example of how to calculate present value; you can use a present value calculator to double-check the results.
If someone is trying to save $50,000 for a down payment on a home in 5 years, how much would have to be invested at 5% today? Plug these numbers into the present value calculator, and the answer is $39,176.31.
This example differs from the net present value in that the investment is made in something that grows over time, whereas a typical net present value example is made in an investment that generates cash flows over time.
The present value can also be calculated on a future stream of cash flows. In the initial example, we had future cash inflows of $1,300 each year for 5 years. If you add the discounted cash flows in the table above, you’d get an amount of $4,928.02.
This means that you would be indifferent between receiving $1,300 a year for the next 5 years or receiving $4,928.02 today, assuming a discount rate of 10%.
Since these cash flows are worth $4,928.02 today, we would not be willing to pay $5,000 for them today, and this is why the business would not proceed with investing in the project.
This example differs from the net present value in that the initial cash outflow ($5,000 in our example) is excluded from the Present Value calculation but included in the Net Present Value calculation.
What is the Difference Between NPV and IRR?
The internal rate of return (IRR) and the NPV tell the same story, just in a different way. The IRR is the discount rate that turns the NPV to $0. We showed this in the example above when the IRR of 9.435% led to an NPV of $0.
The NPV calculator above will compute the IRR for an initial cash outflow followed by a stream of cash inflows. You can also calculate it using an IRR calculator.
It is set up the same way but without the discount rate. Enter in the initial investment and future cash flows, and the IRR Calculator will compute the IRR. We again see that an initial investment of $5,000 followed by 5 years of $1,300 cash flows computes an IRR of 9.435%.
Both the NPV and IRR tell the same story in that there is a rule that will always indicate when a project should be pursued. We indicated earlier that when the NPV is negative, the project should not be undertaken. When the NPV is positive, the company should move forward with the project.
However, we can also determine whether or not a project should be pursued by using the IRR. If the IRR is less than the discount rate, then the company should not pursue the investment. If the IRR is greater than the discount rate, the company should invest in the project.
Every time the NPV is negative, the IRR will be less than the discount rate. And every time the NPV is positive, by definition, the IRR will be greater than the discount rate.
Frequently Asked Questions
Is NPV still used?
NPV is still used today to make investment decisions and determine if an investment is worth pursuing. It is used in economics, accounting, investing, and financial analysis.
When should you use NPV?
NPV is used for various reasons with the main one being to determine if an investment is worth pursuing.
It can also be used for forecasting cash inflows and outflows of a project, analyzing business purchases, making large purchases, such as real estate or equipment, and in startup businesses seeking investors.
Is NPV and DCF the same?
The NPV and DCF are not the same. NPV calculates the present value of future cash flows while discounted cash flow (DCF) calculates the future value of a series of cash flows.
The NPV also deducts the initial investment from its calculation whereas the DCF does not. This could lead to the DCF calculations misrepresenting whether a potential investment is viable or not.
Can NPV be negative?
Yes. A negative NPV shows that a potential investment will not be profitable. When the NPV is negative, this usually means it’s not a good investment and should be avoided, however, some companies will take on projects with a NPV of $0 or that’s negative for various reasons.
A company may take on a project with a negative NPV due to the sunk cost fallacy, because it has a very high discount rate, or to please shareholders if the company is not doing well.