IRR Calculator – Find the Internal Rate of Return
Calculate the internal rate of return (IRR) for an investment using the calculator below.
Results:
| Internal Rate of Return (IRR): | %
|
| Return on Investment (ROI): | %
|
| Net Cash Flow (NCF): | $ |
On this page:
Joseph Rich holds a Master's degree in finance and a Bachelor's degree in economics. He specializes in economics and investing analysis.
Monica Greer holds a PhD in economics, a Master's in economics, and a Bachelor's in finance. She is currently a senior quantitative analyst and has published two books on cost modeling.
Laura started her career in Finance a decade ago and provides strategic financial management consulting. She has an MBA in Finance and a Bachelor's in Economics.
How to Calculate Internal Rate of Return
The internal rate of return (or IRR) is the rate that sets the net present value (NPV) of a stream of cash flows for a project to $0.[1]
NPV is a tool used in corporate finance to estimate future returns on an investment in dollars. We cover NPV in more detail below, and you can also learn more about it on our NPV calculator.
The higher the IRR, the more financially successful a project is. A project’s IRR needs to be higher than the company’s required rate of return in order for the company to move forward with a project.[2]
The minimum required rate of return, sometimes referred to as the hurdle rate, is usually set at the weighted-average cost of capital (WACC) for the investment.
The IRR is essentially the compounded annual growth rate that a project is expected to earn. If a project has an initial investment of $1,000 and earns a 15% internal rate of return for five years, it is equivalent to earning 15% over five years.
While this 15% is not earned consistently each year, the IRR smooths out the return over the lifetime of the project. Try our ROI calculator to see what the compounded annual growth rate is on an investment.
The IRR assumes that each cash flow is received/paid at the end of the year. This is not a likely assumption but allows for simplicity in the calculation.
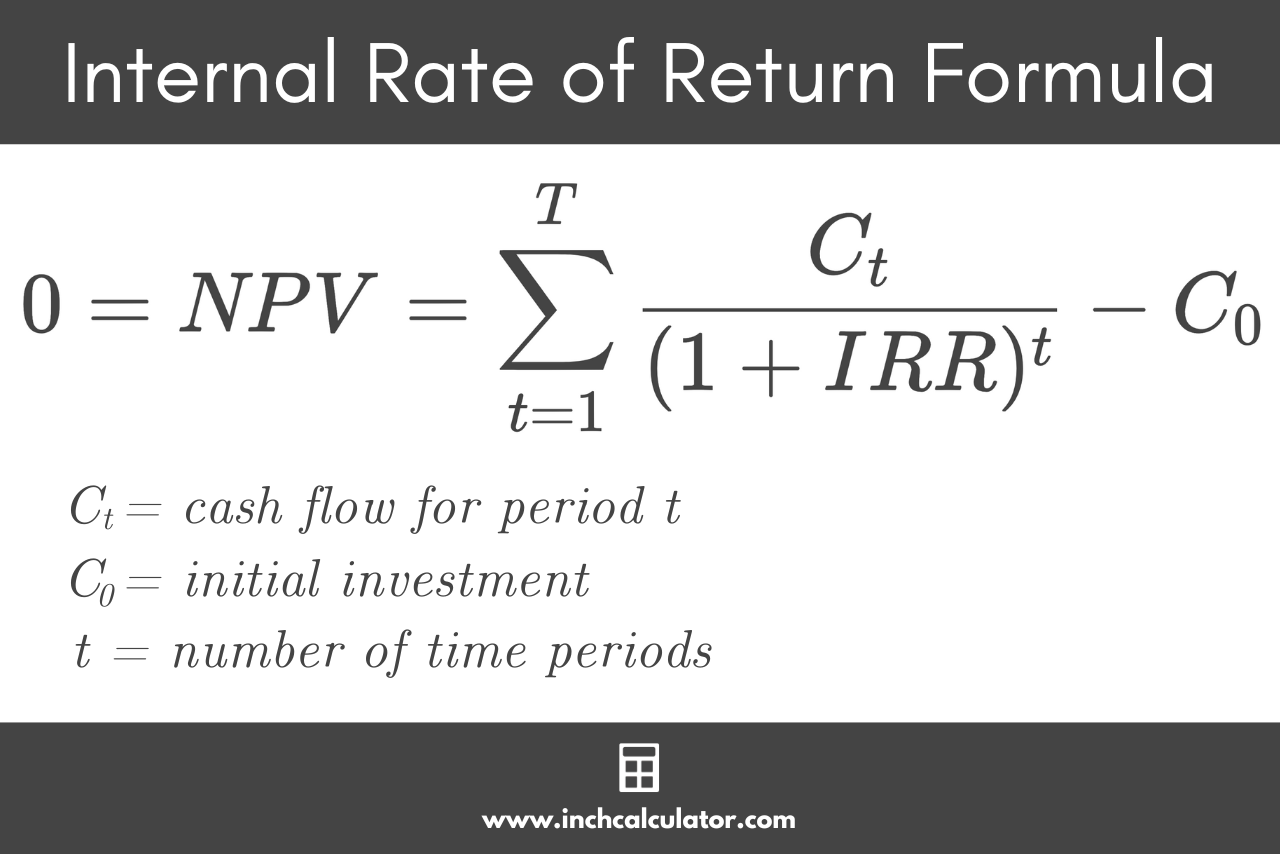
Internal Rate of Return Formula
The following formula expresses the internal rate of return:[3]
where:
NPV = net present value
IRR = internal rate of return
Ct = cash flow for a given period
C0 = initial investment
t = number of time periods
Thus, the internal rate of return is equal to the discount rate where the sum of cash flows divided by the discount rate for each time period, minus the initial investment is equal to zero.
For property investments, you can use our rental property calculator to evaluate the rate of return.
Example of Calculating IRR
It is best to look at a numerical example to see how the IRR works. Let’s say the company is deciding between two projects and has a required rate of return of 12%. This means it won’t accept any project with an IRR under 12%.
The required rate of return is also called the cost of equity found in the WACC calculator. The weighted-average cost of capital calculator shows what a firm’s cost of capital is.
It is a weighted-average of the cost of capital required and the cost of debt. Since the IRR involves investing in a project, the required rate of return needs to equal the company’s cost of capital.
The stream of cash flows for both projects can be found in the table below. We can think of these projects as ones where the company purchases a machine for $10,000 upfront (cash outflow), which provides savings in the future (cash inflows).
| Time | Project A | Project B |
|---|---|---|
| 0 | $(10,000.00) | $(10,000.00) |
| 1 | $6,000.00 | $3,200.00 |
| 2 | $4,000.00 | $2,800.00 |
| 3 | $2,000.00 | $2,400.00 |
| 4 | $1,000.00 | $2,000.00 |
| 5 | – | $1,600.00 |
| 6 | – | $1,200.00 |
| 7 | – | $800.00 |
At first glance, you might think that project B is the winner. They both have an initial $10,000 cash outflow. Project A has $13,000 in total cash inflows, whereas Project B has $14,000 in cash inflows. Additionally, Project B has cash flows for seven years, but Project A only has four years of cash flows.
But, once we plug the numbers into the IRR calculator, we see that Project A has an internal rate of return of 15.86% and Project B has an internal rate of return of 11.79%.
The company can only accept Project A because Project B has an internal rate of return below the company’s 12% required rate of return.
How can this be? How can a project that has $1,000 more in cash inflows and an additional three years of cash inflows have a lower IRR?
There are several reasons for this. The first two involve the time value of money, whereas the third reason calculates the payback period. For a refresher on the time value of money, see our time value of money calculator.
First, the cash flows at the beginning are larger for Project A than for Project B. Since the time value of money states that cash earned now has more value than cash earned in the future, we would prefer the project that has higher cash flows sooner.
So now after year 1, the business can reinvest the $6,000 if the company goes with Project A. If the company chose Project B, it would only have $3,200 to reinvest. After year 2, these numbers are $10,000 and $6,000 for Project A and Project B, respectively.
Since Project A ends sooner, the company can begin reinvesting the funds earlier than it could with Project B.
Second, Project A has a higher net present value. The NPV of Project A is $605, whereas Project B has an NPV of $(54). This is another reason that Project B cannot be accepted. The company would be better off doing nothing and earning an NPV of $0 than investing in a project that has a negative NPV.
In fact, this will always hold true. Anytime a project has an internal rate of return that is below the company’s required rate of return, it will have a negative NPV. Also, anytime a project has an internal rate of return above the company’s required rate of return, it will have a positive NPV.
The table below shows how the NPV is calculated. The sum of column 3 (for Project A) and column 5 (for Project B) calculate their NPVs.
| Time | Project A | Project B | ||
|---|---|---|---|---|
| 0 | $(10,000.00) | $(10,000.00) | $(10,000.00) | $(10,000.00) |
| 1 | $6,000.00 | $5,357.14 | $3,200.00 | $2,857.14 |
| 2 | $4,000.00 | $3,188.78 | $2,800.00 | $2,232.14 |
| 3 | $2,000.00 | $1,423.56 | $2,400.00 | $1,708.27 |
| 4 | $1,000.00 | $635.52 | $2,000.00 | $1,271.04 |
| 5 | – | – | $1,600.00 | $907.88 |
| 6 | – | – | $1,200.00 | $607.96 |
| 7 | – | – | $800.00 | $361.88 |
| Cash Inflows | $13,000.00 | $14,000.00 | ||
| IRR | 15.86% | 11.79% | ||
| NPV | $605.00 | $(53.69) | ||
Third, Project A has a payback period of only two years compared to a payback period of 3.8 years. Project A earns $10,000 after two years which is why its payback period is two years. However, it takes Project B until its fourth year to reach $10,000.
Of course, the payback period here does not take the time value of money into account, you can calculate the payback period accounting for this using our time value of money calculator.
The reason why Project B’s payback period is 3.8 years is that the fourth payment puts the earnings over $10,000, and it is assumed the payment is received evenly throughout the year.
While the lower payback period doesn’t always guarantee a higher NPV or IRR, it does provide a good indicator. Note that this calculation takes the time value of money out of consideration, so it is the least useful of the three reasons.
Note that as mentioned above, this calculation does not take the time value of money into consideration, so it’s not accurate for that purpose, but it is a good indicator when making comparisons.
Overall, these reasons point to why Project A is preferable to Project B, even though Project A has fewer total cash flows and ends three years earlier than Project B.
When the internal rate of return is greater than the required rate of return, it will lead to a positive net present value.
What is the Difference Between IRR and Net Present Value (NPV)?
NPV is another tool used in corporate finance and capital budgeting to value a potential investment opportunity. It is similar to the present value but subtracts the initial investment at the end.
While the IRR is a rate of return, NPV is a dollar amount.
The NPV formula looks like this:
Note that this is similar to the formula to calculate IRR above, but in this case the NPV is not required to equal $0.
The IRR is a rate of return, but the NPV is a dollar amount. In both cases, the higher the amount, the better. They both tell the same story.
If a company calculates a negative NPV on a project, it should avoid it. It can do nothing and earn an NPV of $0, which is higher than a negative value. If the NPV comes to exactly $0, then the company will be indifferent between investing in the project and not investing in the project.
In both cases, its NPV is $0. If the project has a positive NPV, the company should move forward with the project.
The company could also use the IRR to judge potential investment opportunities. If the IRR is less than the required rate of return, then the company should not pursue the investment.
If the IRR is equal to the required rate of return, the company will be indifferent between investing or not investing. Finally, if the IRR is greater than the required rate of return, the company should invest in the project.
Frequently Asked Questions
What does the IRR tell you?
The internal rate of return (or IRR) is the rate that sets the net present value (NPV) of a stream of cash flows for a project to $0. This allows potential investors to estimate the profitability of an investment as a percentage and determine if it is worth moving forward with or not.
Why is IRR used more than NPV?
NPV is similar to the IRR, but it is a dollar amount whereas the IRR is a percentage rate of return. IRR is used more frequently than NPV, because it’s simpler and easier to compare multiple potential investments, especially if you don’t have the discount rate.
NPV is a more commonly used estimate when trying to find the cash flow of a potential investment over time.
How is IRR different from CAGR?
IRR and CAGR are both used to estimate the profitability of a potential investment, however, the IRR includes the potential cash inflows and outflows while the CAGR calculates the annual growth rate with compounding taken into effect only using the beginning and ending value.
References
- Kierulff, H., IRR: A Blind Guide, American Journal Of Business Education, July/August 2012, 5(4), 417-426. https://files.eric.ed.gov/fulltext/EJ1056227.pdf
- Heisinger, K., Ben Hoyle, J., Accounting for Managers - 8.3 The Internal Rate of Return, https://2012books.lardbucket.org/books/accounting-for-managers/s12-03-the-internal-rate-of-return.html
- Wendorf, M., How To Calculate Internal Rate Of Return (IRR), Seeking Alpha, July 12, 2022, https://seekingalpha.com/article/4520014-irr-formula-calculation