APR Calculator
Calculate the annual percentage rate using our APR calculator.
Results:
Annual Percentage Rate (APR)
| Monthly Payment: | $ |
| Amount Financed: | $ |
| Total Payments: | $ |
| Total Finance Charges: | $ |
| Total Interest: | $ |
| Total Fees: | $ |
On this page:
Joseph Rich holds a Master's degree in finance and a Bachelor's degree in economics. He specializes in economics and investing analysis.
Laura started her career in Finance a decade ago and provides strategic financial management consulting. She has an MBA in Finance and a Bachelor's in Economics.
How to Calculate the Annual Percentage Rate
The annual percentage rate (APR) is the simple interest rate combined with other fees that the bank may charge you, such as financing fees and prepaid fees. It represents the true cost of borrowing money if a loan is a simple interest loan.
If you are taking out a loan with a relatively high interest rate but low fees, you may end up with a lower APR than a loan with a low interest rate but high fees. This is why it is recommended to compare the APR between two financial institutions as opposed to comparing only the interest rates.
The APR calculator or the APR formula below provides an easy way to do this.
APR Formula
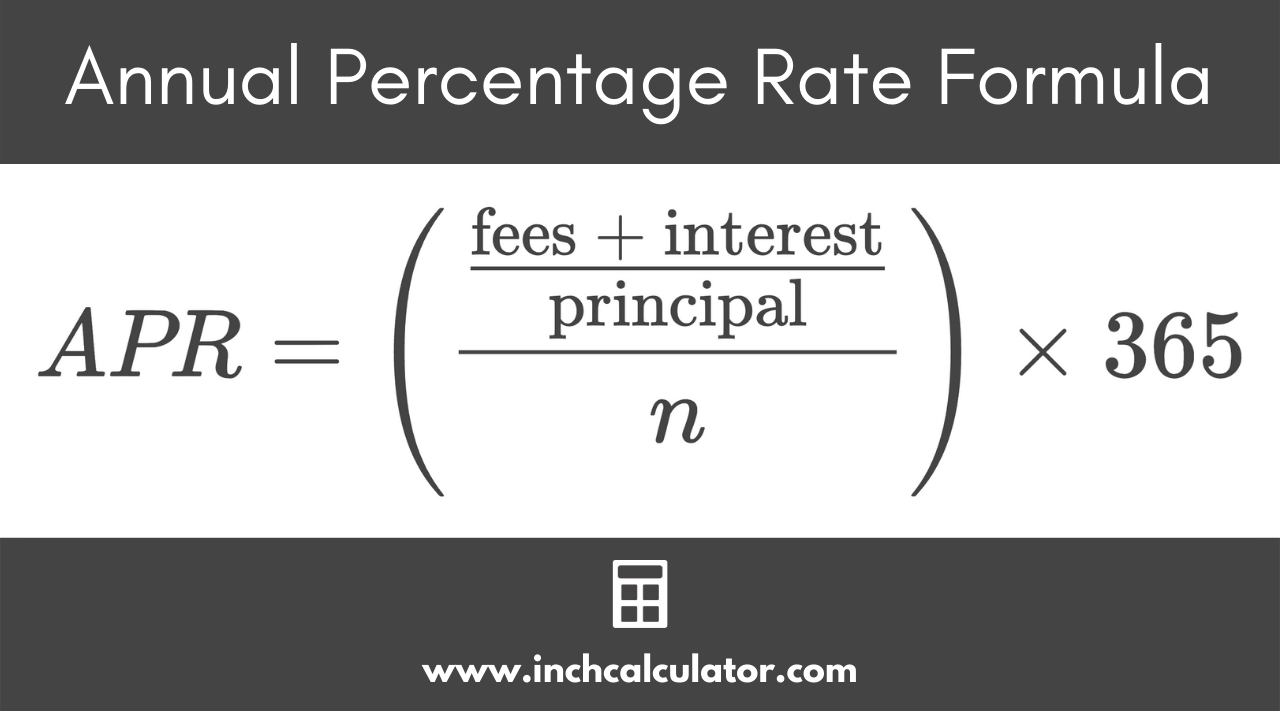
You can use the following formula to calculate an annual percentage rate.
Where:
fees = total fees paid on the loan
interest = total interest paid
principal = initial loan amount
n = loan term in days
Note that the calculator uses the Newton-Raphson method for calculating APR, and it accounts for regular interest payments, so the APR results will differ between the APR formula and the APR calculator.
For example, let’s assume that you are taking out a $5,000 loan that has a 6% interest rate for 2 years. The bank has already notified you that the financing fees will be $200. What will the APR be on this loan?
Before we can use the APR formula above, we should first find what the total interest paid is. We will need to use our simple interest formula below to calculate the total interest.
where:
i = interest
PV = present value
r = interest rate
t = number of periods
Now that we have the total interest, we have everything we need to find the APR:
When shopping for different loans, it is recommended that you compare the APR between them instead of just the interest rate because the APR represents the true cost of borrowing on a loan.
Let’s look at the following examples to see how we might compare two loans.
You are looking for a $10,000 auto loan for 4 years. Bank A has quoted you a 5% loan with $100 in financing fees, whereas Bank B is willing to offer you a 4% loan but with $600 in financing fees. How can you know which loan would be a better option? We need to compare the APR for both and see which offers a lower APR.
But, again, we first need to calculate the simple total interest so we can plug them into the APR formula.
Interest for Bank A:
Interest for Bank B:
Now that we know the total interest, let’s calculate the APR for each loan.
APR for Bank A:
APR for Bank B:
Since Bank A has the lower APR, this is the loan that is more desirable. This is true even though Bank A has a higher interest rate.
Another way to tell which is better is to compare the interest and financing fees. Bank A charged you $2,000 in interest and $100 in financing fees, for a total of $2,100. Whereas Bank B charged you $1,600 in interest and $600 in financing fees, which totals $2,200.
This again shows that Bank A is preferable to Bank B.
Banks are required to disclose the APR of each loan so that hidden fees do not hit you by surprise.
Annual Percentage Rate Explained
APR on Loans
The APR on loans (with the exception of credit cards as discussed in the next section) is exactly what we have calculated above. The APR includes the total interest and all financing charges.
All else being equal, the riskier the loan, the higher the interest rate and APR will be. For example, auto loans will have a higher interest rate than mortgages because the borrower is more likely to miss payments on their car than their house. Either case is bad, but it is better to have the bank take your car than your house.
The financing fees associated with auto loans are origination fees, processing fees, points paid toward the loan, and dealership fees. You can use our auto loan calculator to calculate the payment or the maximum price you can afford.
Mortgages have similar financing fees, but do have a few extra. For mortgages, some of the financing fees are origination fees, processing fees, points paid toward the loan, mortgage insurance, closing costs, and mortgage broker fees.
Loan Payment Formula
You can calculate the payment for a loan using the following formula.
Where:
PMT = monthly payment
P = loan principal
r = annual interest rate
n = number of compounding periods per year
Y = loan term in years
APR on Credit Cards
For credit cards, APR and the interest rate are essentially the same thing, which is unique to credit cards. Even though there are other borrowing costs with credit cards, these are not included in the credit card APR.
The interest rate on a credit card will typically be the highest of any type of loan because credit cards are the riskiest type of loan. If you stop making payments on your credit card, the bank has no collateral to take.
However, if you stop paying your mortgage or auto loan, then the lender is able to legally take the house or car, sell it, and recoup as much of the loan as possible. The credit card lender knows this and needs to be compensated for the additional risks from credit cards.
Also unique to credit cards is that it is possible to never pay interest on it. If you pay off the balance in full each month, you won’t pay any interest. Because credit cards have high APR rates, it is advisable to pay off the balance each month.
However, if you are unable to pay off the balance in full, make sure to watch out for introductory APR rates. Some lenders will introduce a low APR for a certain period, and then increase the APR over time.
Types of APRs
The most common APR is the purchase APR. This is the rate that will be paid on any purchases that are not paid off in full at the end of the month.
As previously mentioned, there can be an introductory APR a lender will give you that increases after a certain period of time.
A third APR is the penalty APR. This is the new rate that would apply if you misused the card such as failing to pay on time. This will be the highest of the types of APRs.
There is also a distinction between fixed and variable APR. Fixed APRs will not change unless you fail to make a payment and incur a penalty. Then the lender can increase the rate. Variable APRs fluctuate as the prime rate does.
The prime rate is the interest rate that banks charge each other for short-term loans. If the prime rate increases, then the credit card rate may also increase.
APR vs. the Nominal Interest Rate
The main difference between the APR and nominal interest rate is that the nominal interest rate does not take the financing or prepaid fees into effect–it is just the simple interest rate.
In our first example, we had a $5,000 loan at 6% for 2 years. We calculated the APR to be 8%. The nominal interest rate was just the 6% that we saw prior to the addition of the financing charges.
APR vs. APY
APY stands for Annual Percentage Yield. APY is the interest rate that is calculated once compounding is taken into effect. With the APY, the more frequent the compounding, the higher the total interest will be.
While interest can technically be compounded at any time frequency, the most common frequencies are daily, monthly, quarterly, semiannually, and annually. These are also available options in our APY calculator.
So, they are similar in that they both annualize the interest rate (and why they both have “annual” in their names).
However, APR and APY differ in several ways:
- The APY takes compounding into consideration, but the APR does not. So the APR more accurately represents the cost of borrowing on a loan because most loans use simple interest. The APY more accurately represents what the return on investment is for a savings account because the bank will pay you compound interest (interest on the principal balance and previously accrued interest).
- The APR includes additional fees that the financial institution may charge on top of the interest rate, whereas the APY only includes the interest rate.
Our APR to APY calculator shows the comparison from the compound to simple interest rate.
You can also use our interest calculator to compare the total interest between simple interest and compound interest.
Frequently Asked Questions
What does APR mean?
APR is the annual percentage rate, which is the simple interest charged on a loan for one year, including financing fees and prepaid fees, that’s usually applied to loans such as mortgages, credit cards, and auto loans.
Does 0% APR mean no interest?
0% APR is usually offered on credit card loans for a certain period of time, on certain types of transactions, or both. It’s best to read the fine print on any 0% APR loan because 0% APR is usually not offered for the life of a loan, otherwise the lender would not be compensated for providing the loan and taking on the risk.
Why is your APR so high with good credit?
Typically, the higher a borrower’s credit score, the lower their APR will be on a loan. However, higher APR could be due to paying less than the monthly payment on a loan or credit card each month, consistently carrying over a credit card balance each month, or it could be used to compensate the lender for offering more significant credit card rewards.
How can you lower your APR?
There are various ways to receive a lower APR that include increasing your credit score, paying off your credit cards each month, not missing monthly loan payments, carrying a low credit card balance, and decreasing your debt to income ratio.